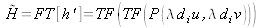Case of a coherent illumination
We showed that (Eq. IV-10),
, i.e :
We define:
-
the spectrum of the input field;
-
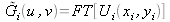the spectrum of the output field;
-
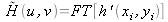the point spread function spectrum.
By applying the convolution theorem:
In what follows, the function
will be called coherent transfer function.
We note that:
therefore:
-
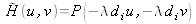
The pupil function P only takes 0 and 1 values: the same is true for the coherent transfer function.
-
There is a finite band in the frequency domain where the diffraction-limited system transmits frequency components without distorting their phase and amplitude. Outside of this band, the frequency response vanishes rapidly.
-
By choosing a coordinate system such that: xi=-xi ; yi =-yi , we can write: